CBSE Sample Papers for Class 12 Applied Mathematics Set 2 with Solutions

Students must start practicing the questions from CBSE Sample Papers for Class 12 Applied Mathematics with Solutions Set 2 are designed as per the revised syllabus.

CBSE Sample Papers for Class 12 Applied Mathematics Set 2 with Solutions

Maximum Marks: 80 Marks
Time Allowed : 3 Hours

General Instructions:

This question paper contains five sections A, B, C, D and E. Each section is compulsory.
Section – A carries 20 marks weightage, Section – B carries 10 marks weightage, Section – C carries 18 marks weightage, Section – D carries 20 marks weightage and Section – E carries 3 case-based with total weightage of 12 marks.
Section A: It comprises of 20 MCQs of 1 mark each.
Section B: It comprises of 5 VSA type questions of 2 marks each.
Section C: It comprises of 6 SA type of questions of 3 marks each.
Section D: It comprises of 4 LA type of questions of 5 marks each.
Section E: It has 3 case studies. Each case study comprises of 3 case-based questions, where 2 VSA type questions are of 1 mark each and 1 SA type question is of 2 marks. Internal choice is provided in 2 marks question in each case-study.
Internal choice is provided in 2 questions in Section – B, 2 questions in Section – C, 2 questions in Section – D. You have to attempt only one of the alternatives in all such questions.

Section – A (20 Marks)

(All questions are compulsory. No internal choice is provided in this section)

Question 1.
The function f(x) = 2x³ + 9x² + 12x – 1 is decreasing on [1]
(a) [-1, ∞)
(b) [-1, 1]
(c) ( – ∞, -2)
(d) [-2, -1]
Solution:
(d) [-2, -1]

Explanation:
Given f(x)= 2x³ + 9x² + 12x – 1
So, f ‘(x) = 6x² + 18x + 12
= 6 (x2 + 3x + 2) = 6 (x + 2) (x + 1)
Here, f ‘(x) ≤ 0 if x [-2, -1]
Hence, f(x) is decreasing on [-2, -1].

Question 2.
The order and degree of the differential equation \(\left(\frac{d s}{d t}\right)^4+3 s \frac{d^2 s}{d t^2}=0\) are respectively: [1]
(a) 2, 3
(b) 1, 2
(c) 2, 4
(d) 2, 11
Answer:
(d) 2, 1

Explanation:
The order of highest order y derivative \(\frac{\mathrm{d}^{2} \mathrm{~y}}{\mathrm{dx}^{2}}\) is 2.
The power of the highest order derivative in the equation is 1.

Question 3.
The area of the region bounded by the curves y² = x and x² = y is: [1]
(a) \(\frac{3}{2}\)
(b) \(\frac{1}{2}\)
(c) 1
(d) 2
Solution:
(b) \(\frac{1}{2}\)

Explanation:
Required:

Question 4.
The value of C for which P(X= n) = C.n³ is the probability function of a random variable X that takes the values 0, 1, 2, 3, 4 is: [1]
(a) \(\frac{1}{10}\)
(b) \(\frac{1}{100}\)
(c) \(\frac{1}{20}\)
(d) \(\frac{1}{30}\)
Solution:
(b) \(\frac{1}{100}\)

Explanation:
Here,
P(X = 0) = C.0³, P(X = 1) = C1³, P(X = 2) = C.2³,
P(X = 3) = C.3³, P(X = 4) = C4³
Further, we know that ΣP(X = x_i) = 1
So, C.0³ + C.1³ + C.2³ + C.3³ + C.4³ = 1
⇒ C(1 + 8 + 27 + 64) = 1
⇒ C = \(\frac{1}{100}\)

Question 5.
The slope of the tangent to the curve x = t² + 3t- 8, y = 2t² – 2t – 5 at the point (2, -1) is: [1]
(a) \(\frac{22}{7}\)
(b) \(\frac{-6}{7}\)
(c) \(\frac{7}{6}\)
(d) \(\frac{6}{7}\)
Solution:
(d) 7

Explanation:
Given x = t² + 3t – 8, y = 2t² – 2t – 5,
we have \(\frac{\mathrm{dx}}{\mathrm{dt}}\) = 2t + 3; \(\frac{\mathrm{dy}}{\mathrm{dx}}\) = 4t – 2
⇒ \(\frac{\mathrm{dy}}{\mathrm{dt}}\) = \(\frac{d y / d t}{d x / d t}\) = \(\frac{4 t-2}{2 t+3}\) ………………. (1)
At (2, -1), we have t² + 3t – 8 = 2 and 2t² – 2t – 5 = -1
⇒ t = 2
Substituting this value of t in (1), we have \(\frac{\mathrm{dy}}{\mathrm{dx}}\) = \(\frac{6}{7}\)
⇒ slope of the tangent to the curve at (2, -1) is \(\frac{6}{7}\).

Question 6.
Max. Z = 4x + y, subject to x + y ≤ 50, 3x + y ≤ 90, x, y ≥ 0 occurs at [1]

(a) (0, 50)
(b) (20, 30)
(c) (50, 0)
(d) (30, 0)
Solution:
(d) (30, 0)

Explanation:
The corner points of the feasible region of the given LPP are (0, 0), (30, 0), (20, 30) and (0, 50). Also, Z_max = 120 at (30, 0)

Question 7.
If the mean and variance of a binomial distribution are 13.5 and 3.375 respectively, then the number of trials is: [1]
(a) 5
(b) 6
(c) 9
(d) 18
Solution:
(d) 18

Explanation:
Here, np = 13.5 and npq = 3.375
So, q = \(\frac{1}{4}\) and hence p = \(\frac{3}{4}\) and n = 18
Thus, the number of trials is 18.

Question 8.
In a race of 150 m, A gives b a start of 20 m.What distance will be covered by B? [1]
(a) 90 m
(b) 130 m
(c) 150 m
(d) 160 m
Solution:
(b) 130 m

Explanation:
Distance covered by B = 150 – 20
= 130 m

Question 9.
If in a binomial distribution n = 600, p = \(\frac{2}{5}\), then its standard deviation is: [1]
(a) 12
(b) 10
(c) 8
(d) 4
Solution:
(a) 12

Explanation:
Here, np = 600 × \(\frac{2}{5}\) = 240 and q = 1 – p = 1 – \(\frac{2}{5}\) = \(\frac{3}{5}\)
So, npq = 240 × \(\frac{3}{5}\) = 144
Thus, S. D = \(\sqrt{n p q}\) = 12

Question 10.
A man purchases a lottery ticket, in which he may win the first prize of ₹ 1,00,000 with probability 0.0001 or the second prize of ₹ 40,000 with probability 0.0004. His mathematical expectation is: [1]
(a) 26
(b) 25
(c) 23
(d) 24
Solution:
(a) 26

Explanation:
E(X) = (1,00,000 × 0.0001) + (40,000 × 0.0004)
= 10 + 16, i.e, 26
So, his expectation is ₹ 26.

Question 11.
If for a Poisson variate X, P(X = 2) = 3 P(X = 3), then the mean of X is: [1]
(a) \(\frac{1}{4}\)
(b) 1
(c) \(\frac{1}{2}\)
(d) \(\frac{1}{3}\)
Solution:
(b) 1

Explanation:
For a P.D,
we have P(X = r + 1) = \(\frac{\lambda}{r+1}\) P(X = r) …………….. (1)
Since it is given that P(X = 2) = 3 P(X = 3), we have
P(X = 3) = \(\frac{1}{3}\) P(X = 2) …………… (2)
From (1) and (2),
we have λ = 1, i.e., the mean is 1.

Question 12.
A runs 1₹ times as fast as B. If A gives B a start of 30 m, how far must the winning post be so that A and B reach at the same time? [1]
(a) 58 m
(b) 66 m
(c) 70 m
(d) 90 m
Solution:
(c) 70 m

Question 13.
Salient features responsible for the seasonal variation are [1]
(a) whether
(b) festivals
(c) social customers
(d) all the above
Solution:
(d) all the above

Question 14.
The area under the normal curve is [1]
(a) 1
(b) 0.75
(c) 0.5
(d) 0.05
Solution:
(a) 1

Explanation:
The entire area beneath the normal curve is one. A normal random variable X has a 0% chance of equalling any value. The area under the normal carve limited by a and plus infinity is equal to the probability that X is bigger than a.
Hence, the area under the whole normal curve is one.

Question 15.
What kind of progression is made by linear trend values? [1]
(a) Arithmetic progression
(b) Geometric progression
(c) Harmonic progression
(d) none of the above
Solution:
(a) Arithmetic progression

Question 16.
The condition for the time reversal test to hold good with usual notation is: [1]
(a) P_bc × P_cb = 1
(b) P_bc × P_cb = 0
(c) P_bc + P_cb = 1
(d) \(\frac{P_{c b}}{P_{b c}}\) = 1 1
Solution:
(a) P_bc × P_cb = 1

Question 17.
Which of the following is false? [1]
(a) The feasible region of a LPP is always a convex polygon.
(b) In a LPP, the constraints are always given by the inequalities.
(c) The minimum value of an objective function Z = ax + by in a LPP always occurs only at the corner point of the feasible region.
(d) If the feasible region of a LPP is bounded, then the objective function Z = ax + by has both maximum and minimum
Solution:
(c) The minimum value of an objective function Z = ax + by in a LPP always occurs only at the corner point of the feasible region.

Question 18.
\(\int \frac{x^2+1}{x^2-1}\) dx is equal to [1]
(a) x + log \(\left|\frac{x-1}{x+1}\right|\) + C
(b) x + log log \(\left|\frac{x-1}{x+1}\right|\) + C
(c) log log |(x – 1) (x + 1)| + C
(d) log |x² + 1| + C
Solution:
(a) x + log \(\left|\frac{x-1}{x+1}\right|\) + C

Explanation:

Assertion-Reason Questions

Two statements are given-one labelled Assertion (A) and the other labelled Reason (R). Select the correct answer to these questions from the codes (a), (b), (c) and (d) as given below:
(a) Both (A) and (R) are true and (IQ is the correct explanation of (A).
(b) Both (A) and (R) are true but (R) is not the correct explanation of (A).
(c) (A) is true but (R) is false.
(d) (A) is false but (R) is true.

Question 19.
Assertion (A): The intermediate solutions of constraints must be checked by substituting them back into objective function. [1]
Reason (R) :

Here, (0, 2); (0, 0) and (3, 0) all are vertices of feasible region.
Solution:
(d) (A) is false but (R) is true.

Explanation:
The intermediate solutions of constraints must be checked by substituting them back into the constraint equation.

Question 20.
Assertion (A): If the matrix P = \(\left[\begin{array}{ccc}
0 & 2 b & -2 \\
3 & 1 & 3 \\
3 a & 3 & 3
\end{array}\right]\) is a symmetric matrix, then a = \(\frac{-2}{3}\) and b = \(\frac{3}{2}\)
Reason (R): If P is a symmetric metrix then P’ = -P
Solution:
(c) (A) is true but (R) is false.

Explanation:
If P is a symmetric matrix, then P’ = P
Hence, R is false.
As P is a symmetric matrix, P’ = P
∴ \(\left[\begin{array}{ccc}
0 & 3 & 3 a \\
2 b & 1 & 3 \\
-2 & 3 & -1
\end{array}\right]=\left[\begin{array}{ccc}
0 & 2 b & -2 \\
3 & 1 & 3 \\
3 a & 3 & -1
\end{array}\right]\)
∴ By equality of matrices, a = \(\frac{-2}{3}\) and b = \(\frac{3}{2}\).

Section – B (10 Marks)

(All questions are compulsory. in case of internal choice, attempt any one question only)

Question 21.
Graph the following system of constraints and shade the feasible region: [2]
3y – x ≤ 10; x + y ≤ 6; x – y ≤ 2
x ≥ 0, y ≥ 0
Solution:

Question 22.
In a game, A can give 15 points to B and 30 points to C. Further, B can give 20 points to C. How many points make the game? [2]
OR
Two pipes P and Q can fill a cistern in 12 minutes and 15 minutes respectively. If both pipes are opened together and at the end of 3 minutes the first pipe is closed, then how much longer will the cistern take to fill the cistern?
Solution:
Let ‘x’ points make the game. Then, A scores x points, B scores (x – 15) points and C scores (x – 30) points B scores x points, C scores (x – 20) points Thus, (x – 15)/(x – 30) = x/(x – 20) x = 60

Part of the tank filled in by both pipes in 1 minute = 1/12 + 1/15 = 3/20
So, Part of the tank filled in by both pipes in 3 minutes = 9/20
Remaining part of the tank that is to be filled = 1 – 9/20 = 11/20
Let the remaining part of the tank be filled in ‘n minutes. Then,
n × 1/15 = 11/20
n = 33/4 , i.e., 8 1/4 minutes.

Question 23.
Find X and Y, if 2X + 3Y = \(\left[\begin{array}{ll}
2 & 3 \\
4 & 0
\end{array}\right]\) and 3X + 2Y = \(\left[\begin{array}{cc}
-2 & 2 \\
1 & -5
\end{array}\right]\) [2]
OR
If A = \(\left[\begin{array}{cc}
1 & 0 \\
-2 & 3
\end{array}\right]\), then prove that,
(A) A + A^T is a symmetric matrix
(B) A – A^T is a skew symmetric matrix
Solution:

OR

Therefore, A + A^T is a symmetric matrix

which is negative of (A – A^T)
Therefore, A – A^T is a skew symmetric matrix.

Question 24.
A shoemaker company produces a specific model of shoes having 15 month average lifetime. One of the employees in their R. D. division claims to have developed a product that lasts longer. This latest product was worn by 30 people and lasted on average for 17 months. The variability of the original shoe is estimated based on the standard deviation of the new group which is 5.5 months. Is the designer’s claim of a better shoe supported by the findings of the trial? Make your decision using two-tailed testing using 5% level of significance. (Given t₂₉(0.05) = 2.05) [2]
Solution:
Here.
H₀: µ = 15, s = 5.5, n = 30 and \(\overline{\mathrm{X}}\) = 17
To test H₀, the statistic t is
t = \(\frac{\bar{X}-\mu}{s / \sqrt{n-1}}=\frac{17-15}{5.5 / \sqrt{29}}\) = 1.96
The table value of t at α = 1105 and 29 d.f. is 2.05.
Conclusion: Since |t| < t_α, the null hypothes is accepted.

Question 25.
Mr. Anup creates an endowment fund of ₹ 60,000 to provide a prize at the starting of every year. This fund earns interest of 8% per annum, compounded annually. What is the prize amount? [2]
Solution:
Here, we have i = 008 and P_☐ = 60,000
Using the formula P_☐ = (R + \(\frac{\mathrm{R}}{i}\) ) we need to determine the vaLue of R.
Using the given values in 60.000
= ₹ (R + \(\frac{\mathrm{R}}{0.008}\))
we have
R = 60,000 × \(\frac{8}{108}\) = 4444.44
Thus, the prize amount is ₹ 4444.44.

Section – C (18 Marks)

(All questions are compulsory. In case of internal choice, attempt any one question only)

Question 26.
Solve the following LPP graphically using comer point method: [3]
Maximise Z = 5x + 7y
subject to the constraints
x + y ≤ 4; 3x + 8y < 24; 10x + 7y < 35 x ≥ 0, y ≥ 0
OR
Solve the following LPP graphically using comer point method:
Minimise Z = x -5y + 20
subject to the constraints
x – y ≥ 0; -x + 2y ≥ 2; x ≥ 3, y ≤ 4 x ≥ 0, y ≥ 0
Solution:
Here, the graph of the given constraints and the feasible region, so obtained, is shaded in the graph given below:

Corner point	Corresponding value of Z
O (0, 0)	5(0) + 7(0) = 0
A (3, 5, 0)	5(3.5) + 7(0) = 17.5
B (\(\frac{7}{3}\), \(\frac{5}{3}\))	5(\(\frac{7}{3}\)) + 7(\(\frac{5}{3}\)) = \(\frac{70}{3}\) = 23\(\frac{1}{3}\)
C(\(\frac{8}{5}\), \(\frac{12}{2}\)	\(5\left(\frac{8}{5}\right)+7\left(\frac{12}{5}\right)=\frac{124}{5}=24 \frac{4}{5}\)
D(0, 3)	5(0) + 7(3) = 21

Thus, maximum value of Z is 24\(\frac{4}{5}\) which occurs at C(\(\frac{8}{5}\), \(\frac{12}{5}\))

Here, the graph of the given constraints and the feasible region, so obtained, is shaded in the graph given below:

Corner point	Corresponding value of Z
A (3,\(\frac{5}{2}\) )	3 – 5(\(\frac{5}{2}\)) + 20 = \(\frac{21}{2}\)
B (6, 4)	6 – 5 (4) + 20 = 6
C (4, 4)	4 – 5 (4) + 20 = 4
D (3, 3)	3 – 5 (3) + 20 = 8

Thus, minimum value of Z is 4 which occurs at C(4, 4).

Question 27.
Express the matrix \(\left[\begin{array}{ccc}
2 & 3 & 1 \\
1 & -1 & 2 \\
4 & 1 & 2
\end{array}\right]\) as the sum of a symmetric matrix and a skew symmetric matrix. [3]
OR
Using inverse coefficient matrix, solve the x + 2y = 5; y + 2z = 8; 2x + z = 5
Solution:

Thus, \(\frac{1}{2}\) (A + A^T) is a symmetric matrix and \(\frac{1}{2}\) (A – A^T) is a skew symmetric matrix.
Further A = \(\frac{1}{2}\) (A + A^T) + \(\frac{1}{2}\) (A – AA^T)

i.e., \(\left[\begin{array}{ccc}
2 & 3 & 1 \\
1 & -1 & 2 \\
4 & 1 & 2
\end{array}\right]\) = \(\frac{1}{2}\left[\begin{array}{ccc}
4 & 4 & 5 \\
4 & -2 & 3 \\
5 & 3 & 4
\end{array}\right]\) + \(\frac{1}{2}\left[\begin{array}{ccc}
0 & 2 & -3 \\
-2 & 0 & 1 \\
3 & -1 & 0
\end{array}\right]\)
Thus, matrix \(\left[\begin{array}{ccc}
2 & 3 & 1 \\
1 & -1 & 2 \\
4 & 1 & 2
\end{array}\right]\) is expressible the sum of a symmetric matrix and a skew symmetric.

Here, A = \(\left[\begin{array}{lll}
1 & 2 & 0 \\
0 & 1 & 2 \\
2 & 0 & 1
\end{array}\right]\), X = \(\left[\begin{array}{l}
x \\
y \\
z
\end{array}\right]\) and B = \(\left[\begin{array}{l}
5 \\
8 \\
5
\end{array}\right]\)
Further, |A| = 9 ≠ 0
A is non-singular and hence A^-1 exists.

x = 1, y = 2, z = 3 is the required solution of the given system of equations.

Question 28.
A soft -drink company claims that the content of each of its soft-drink bottle is:
300 ml One consumer suspects that the bottles are under filled. He measures the contents of 10 bottles which are as follows:
299.7, 298.1, 298.8, 297.6, 301.1,
297.1, 299.3, 298.3, 300.4, 301.5
Do the data confirms the claim? Assume that the contents of the bottles are normally distributed with a standard deviation of 3.5 mL Test your hypothesis at a 5% level of significance. ( Given t_g (0.05) = 2.26) [3]
Solution:
Here we have to test
H₀ : µ = 300 against H₁ : µ ≠ 300
Let µ be the mean content of the soft- drink bottles and \(\bar{x}\) be the sample mean.
Here, the sample size n = 10 and sample mean \(\bar{x}=\frac{2991.9}{10}\) = 299.19 ml
The statistic t is
t = \(\frac{\overline{\mathrm{X}}-\mu}{\mathrm{S} / \sqrt{n}}=\frac{299.19-300}{3.5 / \sqrt{10}}\) = -0.73
⇒ |t| = 0.73
The table value of t at α = 0.05 and 9 d.f. is 2.263.
Conclusion: Since |t| < t_α, the null hypothesis is accepted. And hence, the data confirms the claim.

Question 29.
An investor is considering to purchase 5 years ₹ 1000 par value bond bearing a coupon rate of 7%. The investor’s required rate of return is 8%. The bond is quoted at ₹ 950. The bond will be redeemed at par value. Advise him.
[Given (1.08)^-5 = 0.6805832008] [3]
Solution:
Here,
F = Face value of the bond = ₹ 1000
n = number of periodic divident payments = 5
i = Annual yield rate = 0.08
R = F × i_d = ₹(1000 × 0.07) = ₹ 70
Since the bond is to be redeemed at par,
C = Redemption price or Maturity value = Face value = ₹ 1000
Let V be the purchase price of the bond. Then,
V = \(\mathrm{R}\left[\frac{1-(1+i)^{-n}}{i}\right]\) + F(1 – i)^-n
= ₹ {70 \(\left[\frac{1-(1+0.08)^{-5}}{0.08}\right]\) + 1000 (1 + 0.08)^-5}
= ₹ {875 [1 – (1.08)^-5] + 1000 (1.08)^-5}
= ₹ {875 [1 – 0.6805832008] + 1000 (0.6805832008)
= ₹ {279.49 + 680.58}, or ₹ 960.07
Hence, It is advisable to purchase the bond at ₹ 950.

Question 30.
A bond has a face value of ?1000 matures in 5 years and its present value is ₹ 1200. If coupon rate is 8% p.a. paid semi-annually, find the yield to maturity (YTM) [3]
Solution:
Given, F = ₹ 1000 and i_d = 0.04
R = F × i_d = ₹ (1000 × 0.04) = ₹ 40
Also, Present value = ₹ 1200
Now,
Approx. YTM = Approx. YTM = \(\frac{C+\frac{F-P . V .}{n}}{\frac{F-P . V .}{2}}\)
= ₹ \(\left[\frac{40+\frac{1000-1200}{10}}{\frac{1000+1200}{2}}\right]\) = \(\frac{40-20}{1100}=\frac{2}{110}\) = 0.0182
So, Approx. YTM = 0.0182 or 1.82% per half year or 3.64% per annum
Hence, the yield to maturity is 3.64% per annum.

Question 31.
If a, b and c are any three positive real numbers, then show that (a + b + c) \(\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\) ≥ 9 [3]
Solution:
Consider
(a + b + c) \(\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\) – 9
= \(\frac{c(a-b)^2+a(b-c)^2+b(c-a)^2}{a b c}\)
Since a > 0, b > 0, c > 0, abc > 0 and
(a – b)² ≥ 0 (b – c)² ≥ 0 and (c – a)² ≥ 0
So, \(\frac{c(a-b)^2+a(b-c)^2+b(c-a)^2}{a b c}\) ≥ 0
This gives (a + b + c) \(\left(\frac{1}{a}+\frac{1}{b}+\frac{1}{c}\right)\) ≥ 9

Section – D (20 Marks)

(All questions are compulsory. In case of internal choice, attempt any one question only)

Question 32.
Using integration, find the area of the triangular region whose vertices are [5]
A(-1, 0), B(1, 3) and C (3, 2)
OR
The demand function for a commodity is given by p = 35 – 2x – X², where p and x are respectively the price and the quantity of a commodity, find the consumer’s surplus (CS.) when
(A) market demand x₀ is 2 .
(B) market price p₀ is 20.
Solution:
Let A(-1, 0) , B(1, 3) and C(3, 2) be the vertices of ∆ABC. Then,
Equation of AB: y – 0 = \(\frac{3-0}{1-(-1)}\) (x + 1).
i.e., y = \(\frac{3}{2}\)(x + 1)
Equation of BC: y – 3 = \(\frac{2-3}{3-1}\) (x – 1)
i.e., y = \(\frac{-1}{2} x+\frac{7}{2}\)
Equation of CA: y – 0 = \(\frac{2-0}{3+1}\) (x + 1),
i.e., y = \(\frac{1}{2} x+\frac{1}{2}\)
Required area = Area of the shaded region

= \(\frac{3}{2}\) . 2 + \(\frac{1}{2}\) . 10 – \(\frac{1}{2}\) . 8,
i.e., 4 sq units

The given demand function is p = 35 – 2x – x²
(A) when x₀ is 2,
p₀ = 35 – 2(2) – (2)² = 27

Hence, consumer’s surplus is 9 \(\frac{1}{3}\)

(B) When Po is 20,35 – 2x₀ – x₀² = 20
⇒ x₀² + 2x₀ – 15 = 0
⇒ (x₀ + 5)(x₀ – 3) = 0
⇒ x₀ = -5 or 3
⇒ x₀ = 3 (∵ x₀ ≠ 5)
So, C.S. = \(\int_0^{x_0} p d x-p_0 x_0\)
= \(\int_0^3\left(35 \quad 2 x-x^2\right) d x\) – (20) (3)
= \(\left[35 x-x^2-\frac{1}{3} x^3\right]_0^3\) – 60
= [105 – 9 – 9 – 0] – 60
= 27
Hence, consumer’s surplus is 27

Question 33.
The population of a village increases continuously at the rate proportional to the number of its inhabitants present at any time. If the population of the village was 20,000 in 1999 and 25,000 in the year 2004, what will be the population of the village in 2009? [5]
Solution:
Let y denote the population at any time t.
From the given condition, we have \(\frac{\mathrm{dy}}{\mathrm{dt}}\) = ky,
where k is constant of proportionality.
⇒ \(\frac{\mathrm{dy}}{\mathrm{y}}\) = k dt
Integrating both sides, we have \(\int \frac{d y}{y}=\int k d t\)
⇒ log y = kt + C ……………….. (1)
Let y₀ = 20000 be the population at t = 0.
Then, from (1), C = log y₀
∴ From (1), we get log \(\left(\frac{y}{y_0}\right)\) = kt ………………… (2)
Now y = 25000 when t = 5. So, by (2),

Thus, the population in 2009 was 31250.

Question 34.
An urn contains 5 white, 7 red and 8 black balls. If four balls are drawn one by one with replacement, what is the probability that (i) all are white? (ii) only 3 are white? (ill) none is white? (iv) at last three are white? [5]
OR
A manufacturer of envelopes knows that the weight of the envelopes is normally distributed with mean 1.9 gm and variance 0.01 gm. Find how many envelopes weighing (A) 2 gm or more (B) 2.1 gm or more can be expected in a given packet of 1000 envelopes.
Solution:
Let p denote the probability of drawing a white ball from an urn containing 5 white, 7 red and
8 black balls, Then, p = \(\frac{5}{5+7+8}\) = 0.25
Therefore, q = 1 – p = 1 – 0.25 = 0.75
Let X denote the number of white balls in 4 draws with replacement. Then, X is a binomial variate with parameters n = 4 and p = 0.25
such that P(X = r) = ⁴C_r (0.25)^r(0.75)^4-r; r = 0, 1, 2, 3, 4
Now, Probability that all are white balls = P(X) = ⁴C₄ (0.25)⁴(0.75)⁰
= 0.25⁴, or \(\frac{1}{256}\)
Probability that only 3 are white balls = P(X = ⁴C₃(0.25)³(0.75)¹ = 4(0.25)³ (0.75)¹, or \(\frac{3}{64}\)
Probability that none is white balls = P(X = 0) = ⁴C₀ (0.25)⁰(0.75)⁴ = ( 0.75)⁴, or \(\frac{81}{256}\)
Probability that at least 3 are white balls = P(X ≥ 3)
= P(X = 3) + P(X = 4)
= \(\frac{3}{64}\) + \(\frac{1}{256}\) { From (i) and (ii)}
= \(\frac{13}{256}\)

According to the problem,
Z = \(\frac{X-65.5}{12}\)
(A) P(46 < X < 68)
= P(-1.63 < Z < 0.21)
= P(0 < Z < 1.63) +P(0 < Z < 0.21) = 0.4484 + 0. 0832 = 0.53 16 Thus, the number of students having weight between 46 and 68 kg is 700 × 0.5316 = 372 (B) P(X > 68) = P(Z > 0.21)
= 0.5 – P(0 < Z < 0.21)
= 0.5 – 0. 0832
= 0.4168
Thus, the number of students having weight more than 68 kg is 700 × 0.4 168 = 292

Question 35.
A company has issued a bond having the face value of ₹ 10,000 paying annual dividends at 8.5%. The bond will be redeemed at par at the end of 10 years. Find the purchase price of this bond if the investor wishes a yield rate of 8%. [5]
[Given (1.08)^-10 = 0.46319349] 5
Solution:
Here,
F = Face value of the bond = ₹ 10,000
n = number of periodic divident payments = 10
i = Annual yield rate = 0.08
R = F × i_d = ₹ ( 10,000 × 0.085) = ₹ 850
Since the bond is redeemed at par,
C = Redemption price or Maturity value = Face value = ₹ 10,000
Let V be the purchase price of the bond. Then,
V = \(R\left[\frac{1-(1+i)^{-n}}{i}\right]\) + F(1 + i)^-n
= ₹ {850 \(\left[\frac{1-(1+0.08)^{-10}}{0.08}\right]\) + 10,000(1 + 0.08^-10)}
= ₹ {10625 [ 1 – (1.08)^-10] 10,000(1.08)^-10}
= ₹ {10625 [1 – 0.46319349] + 10,000(0.46319349)}
= ₹ {5703.57 + 4631.93}, or ₹ 10,335. 50
Hence, the purchase price of the bond is ₹ 10,335.50.
Let x = (1.08)^-10
⇒ log x = – 10 log (1.08)
⇒ log x = – 10 × 034237555
= – 0.334237555
or
⇒ x = anti log ()
= 0.46319349

Section – E (12 marks)

(All questions are compulsory. In case of internal choice, attempt any one question only)

Question 36.
A statement which involves variable (s) and the sign of inequality i.e., >, <, ≥ or ≤ is an inequation or an inequality ax + b < 0, ax + b > 0, ax + b ≤ 0 or are linear inequation in one variable x.
And inequations like ax + by ≤ c, ax + by c or ax + by ≥ c are linear in equations in two variables x and y. And solution of an inequation is the value (s) or the variable (s) that makes it a true statement.
(A) Solve the linear inequation.
– 3x + 12 < 0 [1]
(B) What is the value of x in 5x – 3 < 3x + 1, when x is a natural number. [1]
(C) Solve the following in equations [2]
\(\frac{2 x-3}{y}\) + 9 ≥ 3 + \(\frac{4 x}{3}\)
OR
Solve the following inequation.
\(\frac{2 x+4}{x-1}\) ≥ 5
Solution:
(A) Given, -3x + 12 < 0
-3x < – 12 ⇒ \(\frac{-3 x}{-3}>\frac{-12}{-3}\)
⇒ x > 4
Thus, for any real number greater than 4 is a solution of the given inequation.

(B) We have,
5x – 3 < 3x + 1
⇒ 5x – 3x < 3 + 1
⇒ 2x < 4
⇒ \(\frac{2 x}{2}<\frac{4}{2}\)
⇒ -x < 1
If x ∈ N, then x = 1

(C) We have,
\(\frac{2 x-3}{4}\) + 9 ≥ 3 + \(\frac{4 x}{3}\)
⇒ \(\frac{2 x-3}{4}-\frac{4 x}{3}\) ≥ 3 – 9
⇒ \(\frac{3(2 x-3)-16 x}{12}\) ≥ -6
⇒ \(\frac{6 x-9-16 x}{12}\) ≥ -6
⇒ \(\frac{-9-10 x}{12}\) ≥ -6
⇒ -10x ≥ -72 +9
⇒ -10x ≥ -63
⇒ \(\frac{-10 x}{-10} \leq \frac{-63}{-10}\)
⇒ x ≤ \(\frac{63}{10}\)
x ∈ (-∞, \(\frac{63}{10}\))
Hence, the solution set of the given in equations is (–∞, \(\frac{63}{10}\)).

Question 37.
There are ture families A & B. There are 4 men, 6 women and 2 children in family A and 2 men, 2 women and 4 children in family B.
The recommended daily allowance for calorie in man 2400, woman 1900, child: 1800 and for proteins in man: 55 gm women: 45 g and child: 33 gm.
(A) Represent the numbers of family A & B in the form of a matrix. [1]
(B) Form a matrix for the daily recommended daily allowance of calorie and protein for man, woman and child. [1]
(C) Calculate the total requirement of calories fbr two families. [2]
OR
Calculate the total requirement of proteins for two families.
Solution:

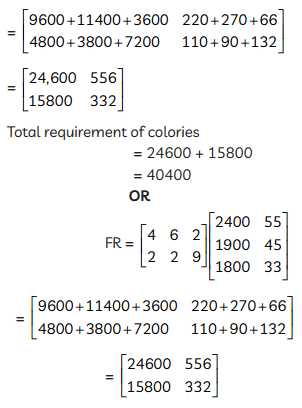
Total requirement of colonies
= 556 + 332
= 888 gm.

Question 38.
Read the following passages and answer the questions that follow:
Let [x] denote the greatest integer, Less than or equal to x and n is a positive integer.
(A) Find the value of [5.79]. [1]
(B) Find the value \(\int_{-n}^n\) [x] dx, for n = 1. [2]
OR
Find the value of \(\int_{-n}^n\) [x] dx, for n = 2.
(C) Find the value of \(\int_{.}^n\) [x] dx, for n = 1. [1]
Solution:
(A) Here. [5.79] = x, where x is an integer such that x ≤ 5.79.
Thus. x = 5.

(B) For n = 1,